eguruchela

List of formulas related to alternating current

i: instantaneous current (A),

Io: maximum instantaneous current (A),

T: period of the wave,

f: frequency,

λ: wavelength,

c: speed of light

../images/Presentation10.jpg

$$ i = i_o cos\omega t $$ $$ f = \frac{1}{T} $$ $$ \omega = 2\pi f $$ $$ \lambda = \frac{c}{f} = cT $$ $$ c \thickapprox 3 \text{ x } 10^8 m/s $$

$$ U = \frac{U_o}{\sqrt{2}} $$ $$ I = \frac{I_o}{\sqrt{2}} $$

$$ U_R = IR $$ $$ u_R = I_o R cos\omega t $$

Ohm's Law of AC Current Containing Only Resistance R

ZL: inductive reactance

$$ u_L = I_o Z_L cos(\omega t + \frac{\pi}{2}) $$ $$ Z_L = L_\omega $$ $$ U_L = IZ_L $$

inductive reactance

ZC: capacitive reactance

$$ u_c = I_o Z_c cos(\omega t - \frac{\pi}{2}) $$ $$ Z_c = \frac{1}{\omega C } $$ $$ U_c = IZ_c $$

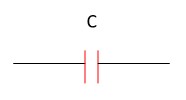
../images/capacitive reactance

Z: impedance

$$ u = I_oZcos(\omega t + \phi) $$ $$ Z = \sqrt{R^2 + (Z_L - Z_C)^2} $$ $$ U = \sqrt{{U_{R}} ^2 + (U_L - U_C)^2} $$

../images/Presentation14.jpg

$$ tan\phi = \frac{U_L - U_C}{U_R} $$ $$ = \frac{Z_L - Z_C}{Z} $$

$$ cos\phi = \frac{U_R}{U} = \frac{R}{Z}$$

$$ P = UIcos\phi = RI^2 $$

$$ \phi = 0 $$ $$ Z_{min} = R $$ $$ I_{max} = \frac{U}{R} $$ $$ Z_L = Z_C $$ $$ \omega = \frac{1}{\sqrt{LC}} $$ $$ cos\phi _{max} = 1 $$ $$ U_L = U_C $$ $$ U = U_R $$

N1, N2: number of turns in the primary coil and secondary coil; U1, U2: primary and secondary voltage; I1, I2: primary and secondary current

$$ \frac{U_1}{U_2} = \frac{N_1}{N_2} = \frac{I_1}{I_2} $$